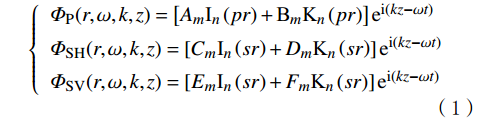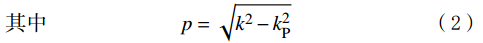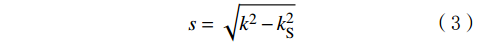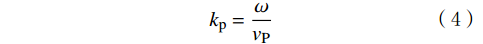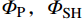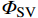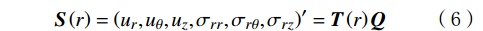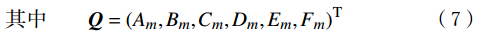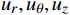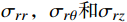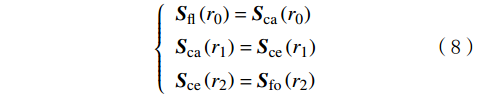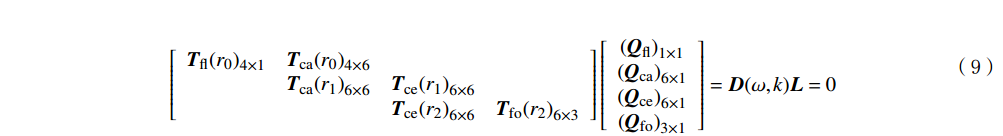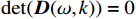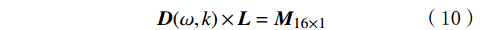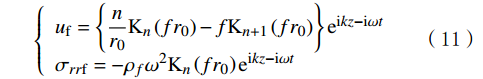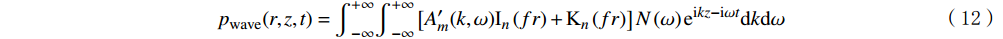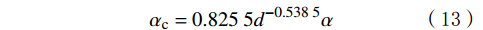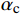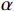Influence of Casing and Cement Sheath Dimensions on Casing Waves in CBL/VDL Logging
-
摘要:
深井超深井厚套管、薄水泥环等复杂井况下利用CBL/VDL测井评价固井质量时,是利用测量的套管波幅度或衰减评价水泥环的胶结状况。但截至目前,对套管中模式波传播机理的研究较少,精细固井质量评价缺乏理论支撑。为此,建立了柱状多层介质的套管井模型,计算得到了套管模式波的相速度、衰减及灵敏度曲线,研究了非胶结因素、套管直径及水泥环尺寸等对套管波衰减的影响。计算结果表明:在CBL/VDL测井频段内存在3~4阶轴向振动模态的套管波,套管外径越大,振动模态越多;套后胶结水泥时,套管波幅度随着套管壁厚增厚明显增大,但自由套管的套管波幅度基本不变;灵敏度曲线表明,套管波衰减对水泥横波速度的灵敏度明显高于纵波,说明单极子声源激发的套管波主要通过剪切耦合向水泥环泄漏能量;另外,地层中纵横波声场快照表明,套管波沿着套管传播时还会向地层中泄漏能量,使水泥环厚度和岩性等的变化也影响套管波幅度。研究结果为复杂井况下固井质量的精细评价及套管波幅度校正图版的绘制奠定了理论基础。
Abstract:When using CBL/VDL logging to evaluate cementing quality under complex well conditions such as thick casing and thin cement sheath in deep and ultra-deep wells, the measured amplitude or attenuation of the casing wave is used to determine the cementation condition of cement sheath. However, the research on the propagation mechanism of the casing mode waves is insufficient, and there is a lack of theoretical support for precise cementing quality evaluation. Therefore, a cased well model with cylindrical multi-layer medium was established, and the phase velocity, attenuation, and sensitivity curves of the casing mode waves were calculated. The effects of non-cementing factors, casing diameter, and cement sheath size on casing wave attenuation were studied. The results show that there are 3~4 order axial vibration modes of the casing wave within the range of CBL/VDL logging frequencies. The larger outer diameter of the casing, the more vibration modes there will be. When the casing is cemented, the amplitude of the casing wave increases obviously as the casing thickness increases, while the amplitude of casing waves basically remains unchanged for free-standing casing. Sensitivity curves show that the attenuation of the casing wave is more sensitive to cement shear wave velocity than to the compressional wave velocity, suggesting that casing wave induced by monopole source leaks energy to cement sheath mainly through shear coupling effect. In addition, the snapshots of shear wave and compressional wave fields show that the casing wave also leaks energy to the formation when propagating along the casing, so the change in cement sheath thickness and lithology will also affect the amplitude of the casing wave. The research results provide a theoretical basis for the precise evaluation of cementing quality nd the creation of calibration chart for the casing wave amplitude in complex well conditions.
-
Keywords:
- casing wave /
- cement sheath thickness /
- dispersion /
- attenuation /
- sensitivity
-
CBL/VDL测井是固井质量检测最主要和最经典的方法,分别利用套管波幅度和地层波幅度对水泥环第Ⅰ界面和第Ⅱ界面进行胶结评价。早期的套管井中声波测井的理论模拟为研究各影响因素提供了理论依据,K.M.Tubman等人[1]建立柱状分层模型,采用在界面间增加流体环的方法模拟套管−水泥界面或水泥−地层界面胶结差的问题,建立了自由套管、第Ⅰ界面胶结差、第Ⅱ界面胶结差和胶结良好的4种经典套管井模型。董庆德等人[2]定性分析了套管与地层之间的胶结状况对套管波形态和幅度的影响,通过分析发现水泥窜槽导致套管波幅度明显降低。陈德华等人[3]给出了在水泥密度和套管尺寸变化时套管波幅度的变化。针对套管井多层结构的分波计算,Zhang Xiumei等人[4]计算了套管井中套管模式波的频散曲线,分析了第Ⅰ界面和第Ⅱ界面不胶结时模式波频散曲线的变化特征,逐渐认识到套管井中测得的套管波可看做是沿着套管轴向传播的多个(纵向)模态的叠加。Wang Huan等人[5]计算了套管井中模式波的频散曲线,提出了可以利用沿套管与地层之间水环传播的斯通利波评价固井质量。国内外学者的研究大多集中在水泥不同胶结状况对套管波响应特征的影响[6–10],但影响套管波幅度的因素除了水泥强度和界面的胶结质量[11–12],还受其他因素的影响,例如套管壁厚和水泥环厚度等也是重要的关键参数,但也容易被忽视。在自由套管状态下套管壁厚的变化对套管波幅度的影响很小,但在胶结水泥后,套管壁厚增厚,井内接收到套管波的幅度明显增大;在深层、超深层套管井中还经常遇到薄水泥环,水泥环厚度小于19.0 mm时套管波幅度或衰减需要校正[10],可见套管波幅度受多种因素的影响。基于此,笔者计算了套管模式波的频散、衰减及灵敏度曲线,分析了套管中模式波的响应特征,给出了不同胶结状态下套管壁厚及水泥环厚度变化引起套管波幅度变化的规律,揭示了CBL/VDL单极子声源激发的套管波衰减的主要影响因素,为下一步开展固井质量的精细评价提供了理论依据。
1. 套管井声场的理论计算方法
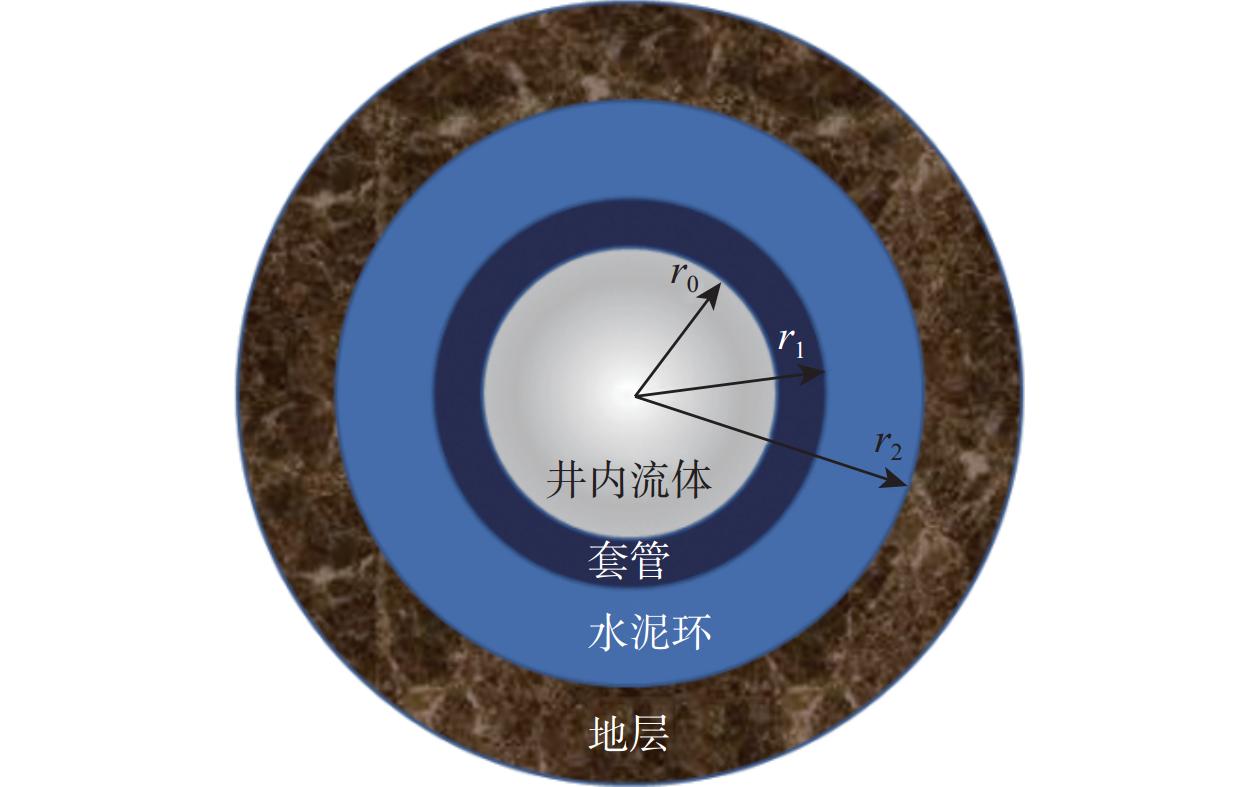
建立柱状分层模型表示套管井模型,以胶结良好模型为例,从内向外的介质分别是井内钻井液、套管、水泥环和无限大地层,套管与水泥环界面为第Ⅰ界面,水泥环与地层界面为第Ⅱ界面,介质边界的半径分别为r0,r1和r2(见图1)。
根据经典弹性波动理论,在柱坐标系中可以求解胶结良好模型中固体介质纵波(P)、横波(SH、SV)的位移势函数,其可以表示为:
{ΦP(r,ω,k,z)=[AmIn(pr)+BmKn(pr)]ei(kz−ωt)ΦSH(r,ω,k,z)=[CmIn(sr)+DmKn(sr)]ei(kz−ωt)ΦSV(r,ω,k,z)=[EmIn(sr)+FmKn(sr)]ei(kz−ωt) (1) 其中p=√k2−k2P (2) s=√k2−k2S (3) kp=ωvP (4) ks=ωvS (5) 式中:
ΦP,ΦSH 和ΦSV 分别为纵波、SH横波和SV横波的位移势函数;r,z分别为套管井的径向和轴向位置,m;k为轴向波数,m−1; ω为角频率,rad/s;t为时间,s;In,Kn分别为第一类和第二类n阶贝塞尔函数;下标n表示环向方位序数,CBL(cement bond log)测井的声源是单极子声源,n=0;Am,Cm和Em为介质中从外向内传播声波的振幅系数,最外侧地层中这3个振幅系数均为0;Bm,Dm和Fm为介质中从内向外传播声波的振幅系数;下标m为套管井模型介质层数;p和s分别称为纵波和横波的径向波数,m−1;kP,kS分别为纵波和横波波数,cm−1;vP,vS分别为介质纵波和横波的速度,m/s。利用位移势函数可以计算弹性介质中的位移与应力分量,得到位移−应力矢量S的方程:
\boldsymbol{S}\left(r\right)={\left({u}_{r},{u}_{\theta },{u}_{z},{\sigma }_{rr},{\sigma }_{r\theta },{\sigma }_{rz}\right)}^{\prime}=\boldsymbol{T}\left(r\right)\boldsymbol{Q} (6) 其中 \qquad\boldsymbol{Q}=({A}_{m},{B}_{m},{C}_{m},{D}_{m},{E}_{m},{F}_{m}{)}^{\mathrm{T}} (7) 式中:
{u}_{r},{u}_{\theta },{u}_{z} 分别为径向、环向和轴向的位移,m;\sigma_{rr},\sigma_{r\theta}和\sigma_{rz} 分别是径向正应力和2个切向应力,Pa;T(r)为6×6系数矩阵。当相邻介质都为固体介质时,为固−固界面,界面处的位移和应力分量连续;当相邻介质分别为固体和液体时,为固−液界面,流体介质中的切应力为0。由此,可得到胶结良好模型的边界条件:
\left\{\begin{array}{l}{\boldsymbol{S}}_\mathrm{fl}\left({r}_\mathrm{0}\right)={\boldsymbol{S}}_\mathrm{ca}\left({r}_\mathrm{0}\right)\\ {\boldsymbol{S}}_\mathrm{ca}\left({r}_\mathrm{1}\right)={\boldsymbol{S}}_\mathrm{ce}\left({r}_\mathrm{1}\right)\\ {\boldsymbol{S}}_\mathrm{ce}\left({r}_\mathrm{2}\right)={\boldsymbol{S}}_\mathrm{fo}\left({r}_\mathrm{2}\right)\end{array}\right. (8) 式中:Sfl,Sca,Sce和Sfo分别为流体、套管和地层界面处的位移和应力矢量矩阵。
将套管井模型各个界面的边界条件联合起来有2种方法,一种是传播矩阵,另一种是全局矩阵法。本文采用全局矩阵法,得到了关于各层介质振幅系数Q的方程:
\begin{gathered} \\ \left[ {\begin{array}{*{20}{c}} {{{\boldsymbol{T}}_\mathrm{fl}}{{({r_0})}_\mathrm{4 \times 1}}}&{{{\boldsymbol{T}}_\mathrm{ca}}{{({r_0})}_\mathrm{4 \times 6}}}&{}&{} \\ {}&{{{\boldsymbol{T}}_\mathrm{ca}}{{({r_1})}_\mathrm{6 \times 6}}}&{{{\boldsymbol{T}}_\mathrm{ce}}{{({r_1})}_\mathrm{6 \times 6}}}&{} \\ {}&{}&{{{\boldsymbol{T}}_\mathrm{ce}}{{({r_2})}_\mathrm{6 \times 6}}}&{{{\boldsymbol{T}}_\mathrm{fo}}{{({r_2})}_\mathrm{6 \times 3}}} \end{array}} \right]\left[ {\begin{array}{*{20}{c}} {{{({{\boldsymbol{Q}}_\mathrm{fl}})}_\mathrm{1 \times 1}}} \\ {{{({{\boldsymbol{Q}}_\mathrm{ca}})}_\mathrm{6 \times 1}}} \\ {{{({{\boldsymbol{Q}}_\mathrm{ce}})}_\mathrm{6 \times 1}}} \\ {{{({{\boldsymbol{Q}}_\mathrm{fo}})}_\mathrm{3 \times 1}}} \end{array}} \right] = {\boldsymbol{D}}(\omega ,k){\boldsymbol{L}} = 0 \\ \end{gathered} (9) 式中:Tfl,Tca,Tce和Tfo分别为井内流体、套管、水泥环和地层的系数矩阵;L为套管井模型各层介质振幅系数组成的列向量。
当系数矩阵行列式
\mathrm{det}(\boldsymbol{D}(\omega ,k))=0 时,可得到套管井中所有模式波的频散曲线;将求解得到的(ω,k)代入式(9),求解齐次方程组,得到各层介质中的振幅系数;利用振幅系数及对应的(ω,k),可以计算各层介质的径向和轴向位移,即为该模式波的波结构。考虑声源的存在,套管井模型的位移−应力求解方程为:
\boldsymbol{D}(\omega ,k)\times \boldsymbol{L}={\boldsymbol{M}}_{16\times 1} (10) 式中, M为与声源辐射的直达波有关的项,其中第一项和第二项为声源辐射声波在井壁处产生的径向位移(uf)与正应力(σrrf),其他项均为0。第一项和第二项的表达式为:
\left\{\begin{array}{l}{u}_{{\mathrm{f}}}=\left\{\dfrac{n}{{r}_{0}}\text{K}_{n}\left(f{r}_{0}\right)-f\text{K}_{n+1}\left(f{r}_{0}\right)\right\}{{\mathrm{e}}}^{{\mathrm{i}}kz-{\mathrm{i}}\omega t}\\ {\sigma }_{rr{\mathrm{f}}}=-{\rho }_{f}{\omega }^{2}\text{K}_{n}\left(f{r}_{0}\right){{\mathrm{e}}}^{{\mathrm{i}}kz-{\mathrm{i}}\omega t}\end{array}\right. (11) 式中,f为流体的径向波数,m−1。
求解式(10),得到各层介质的振幅系数,其中第一项为井筒内流体的响应函数A'n。
利用实轴积分方法,计算井内接收的声压波形:
p_{{\mathrm{wave}}}(r,z,t)={\int }_{-\infty }^{+\infty }{\int }_{-\infty }^{+\infty }\left[{A}_{m}^{\prime}(k,\omega )\text{I}_{n}\left(fr\right)+\text{K}_{n}\left(fr\right)\right]N\left(\omega \right){{\mathrm{e}}}^{{{\mathrm{i}}kz}-{{\mathrm{i}}\omega t}}{\mathrm{d}}k{\mathrm{d}}\omega (12) 式中:pwave为声压,Pa;N(ω)为高斯声源函数。
2. 不同参数条件下套管波响应的计算结果分析
理论计算时套管井模型的声学参数如表1所示,具体数值在分析水泥密度、纵横波速度及地层纵波速度等变化对套管波的影响时会有微调。
表 1 套管井模型中各层介质的声学参数Table 1. Acoustic parameters of each layer medium in cased well model介质 纵波速度/(m·s−1) 横波速度/(m·s−1) 密度/(kg·m−3) 钻井液/水 1 500 1 000 套管 5 959 3 229 7 800 水泥Ⅰ 2 714 1 503 1 300 水泥Ⅱ 2 828 1 729 1 900 地层 3 500 2 000 2 500 2.1 套管波的频散和衰减特征
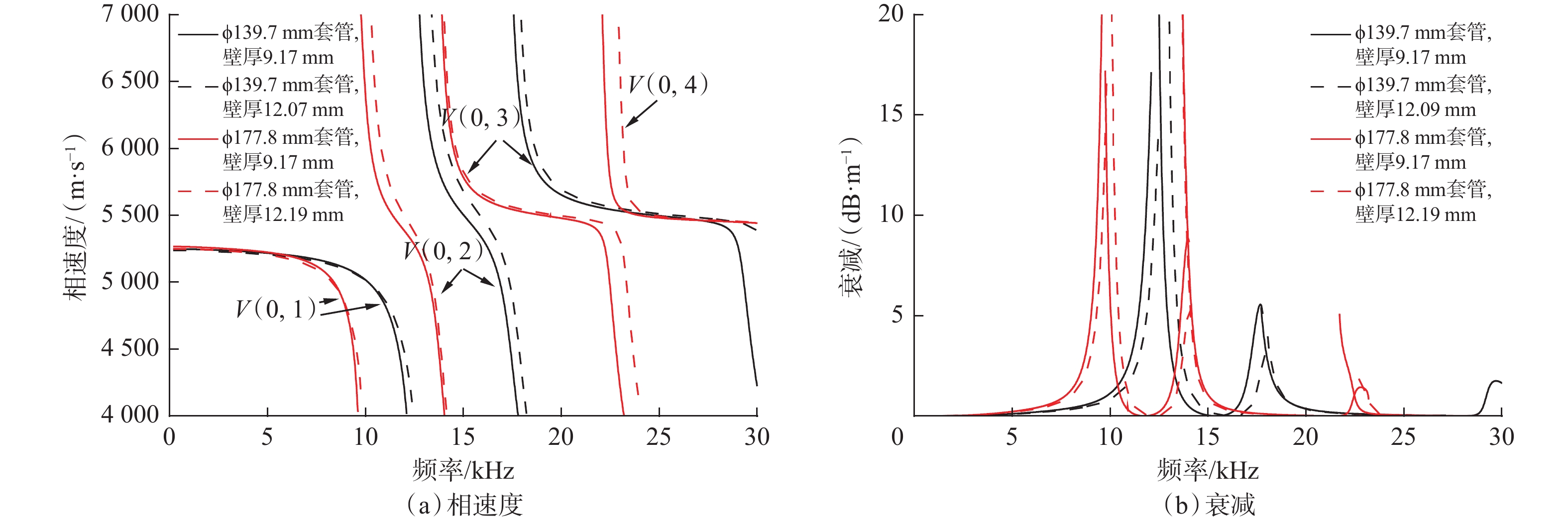
居中的单极子声源在环向胶结均匀的套管井模型中激发的声场是轴对称声场,对于多层结构的套管井,套管中激发的导波是轴对称的纵向模态,质点的振动包括轴向和径向2个方向。套管外径分别为139.7 mm和177.8 mm,水泥环厚度均为20.0 mm,求解式(9)系数矩阵行列式为0的方程,可得到套管模式波的相速度和衰减曲线,如图2所示。从图2可见,随着频率升高,纵向模态的高阶模式会逐渐出现,频率从低到高出现的模态分别设为V(0,1)、V (0,2)、V (0,3)和V (0,4),0代表套管周向阶数为0,整个环向上的声场分布是一致的;1~4分别代表纵向模态的阶数。随着套管外径从177.8 mm减小至139.7 mm,模式波的频散曲线向高频方向偏移,低频段出现模式波的阶数会减少。对于同一外径套管井模型,随着套管壁厚增厚,同一模式的频散曲线也稍稍向高频偏移,但自由套管状态下套管壁厚引起相速度和衰减的变化很小。对于自由套管模型,从模式波的衰减曲线可见,在相速度变化较快的频段,频散引起的耗散衰减较高,但套管波传播引起的泄漏衰减很低。
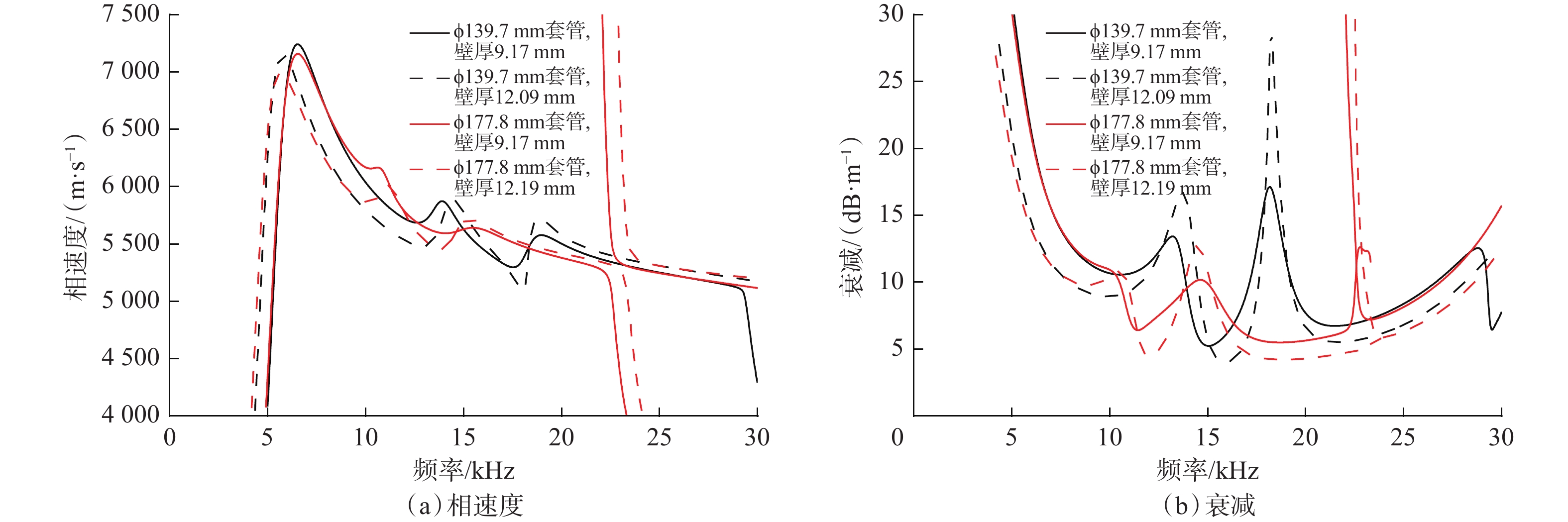
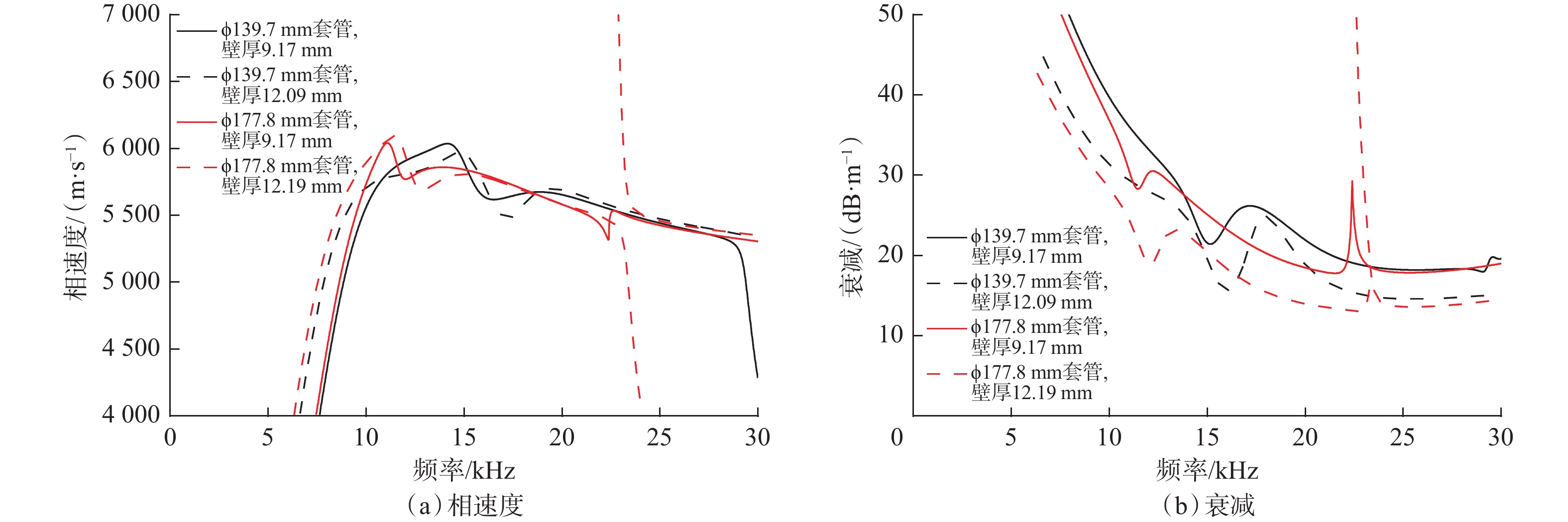
套管后胶结水泥时会明显改变套管波的频散和衰减特征,图3和图4分别为套后耦合1.30 kg/L低密度水泥和1.90 kg/L常规水泥后模式波的相速度和衰减曲线。从图3和图4可以看出,当套管后胶结了水泥时,不同壁厚套管模式波的衰减差异明显增大,壁厚较厚套管模式波的衰减明显降低;随着水泥声阻抗增大,这种变化趋势更加明显。
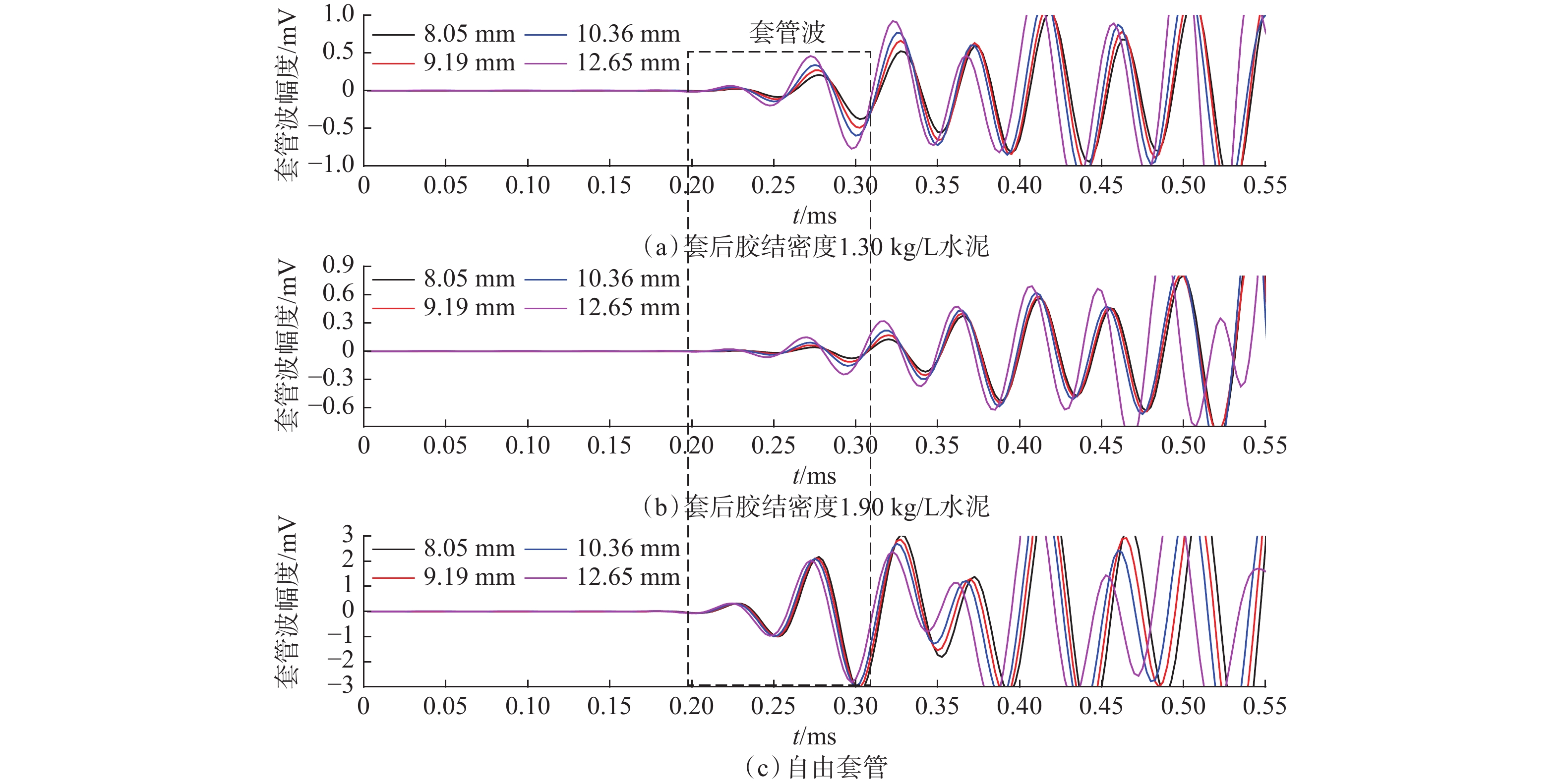
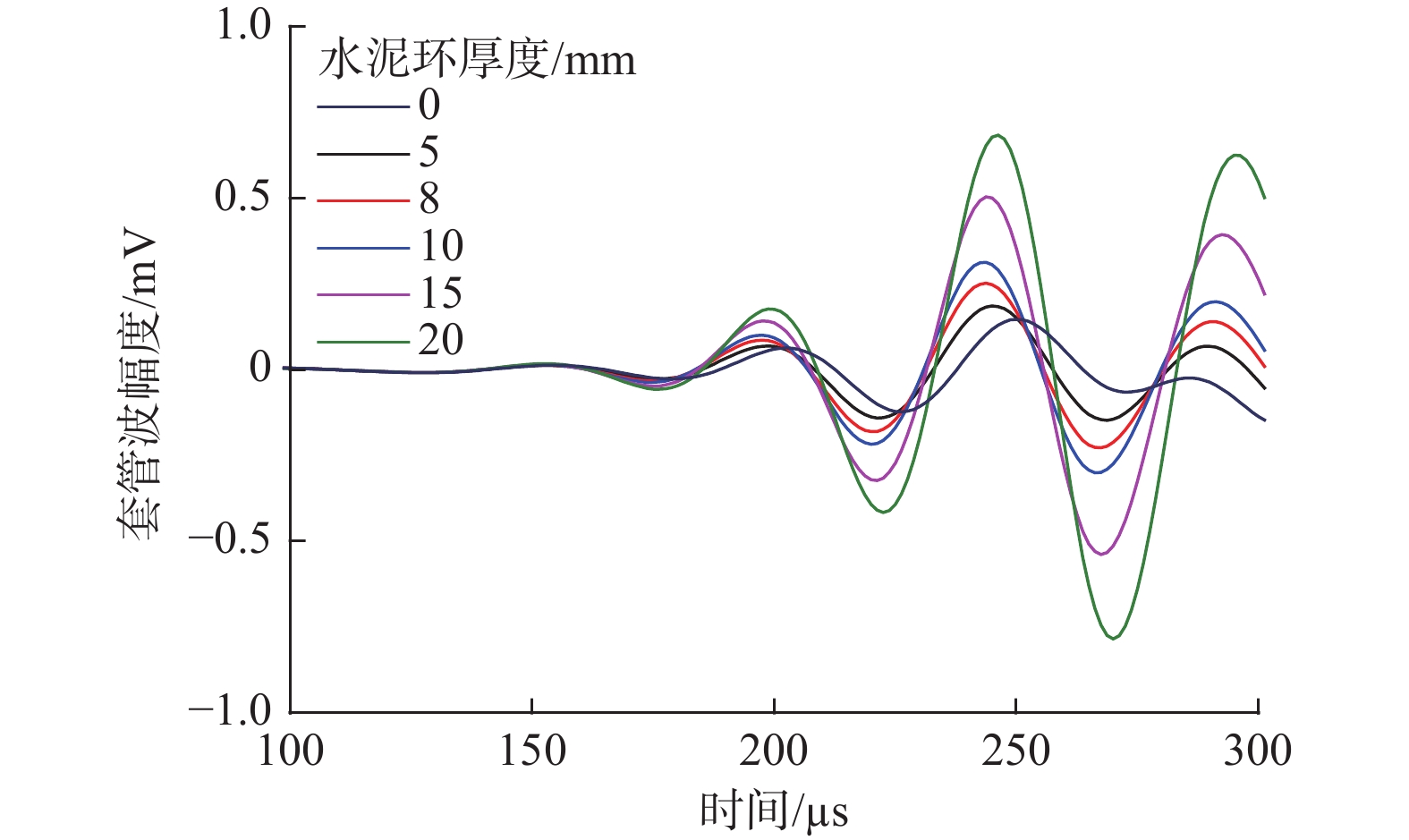
井孔中接收套管波的波形更直观地展现了套管壁厚对套管波幅度的影响,图5为4种套管条件下井孔中接收的全波波形,套管外径均为177.8 mm,可明显看到,随着套管壁厚增厚,在自由套管状态下套管波幅度的变化很小,这与图2显示的自由套管状态下套管波的衰减对壁厚变化不敏感一致;但套后胶结了水泥时,套管波幅度随着套管壁厚增厚明显增大,与图3和图4中套管波衰减降低的变化规律一致。由此可见,壁厚较厚套管在胶结水泥后,套管中的声波能量会向套管内壁集中,使井内接收到套管波的幅度明显增大,套管外壁的声耦合减弱。另外,随着套管壁厚增厚,到时稍有提前,这与此计算主频下群速度稍有增大和套管壁厚增厚导致的套管内径减小有关。
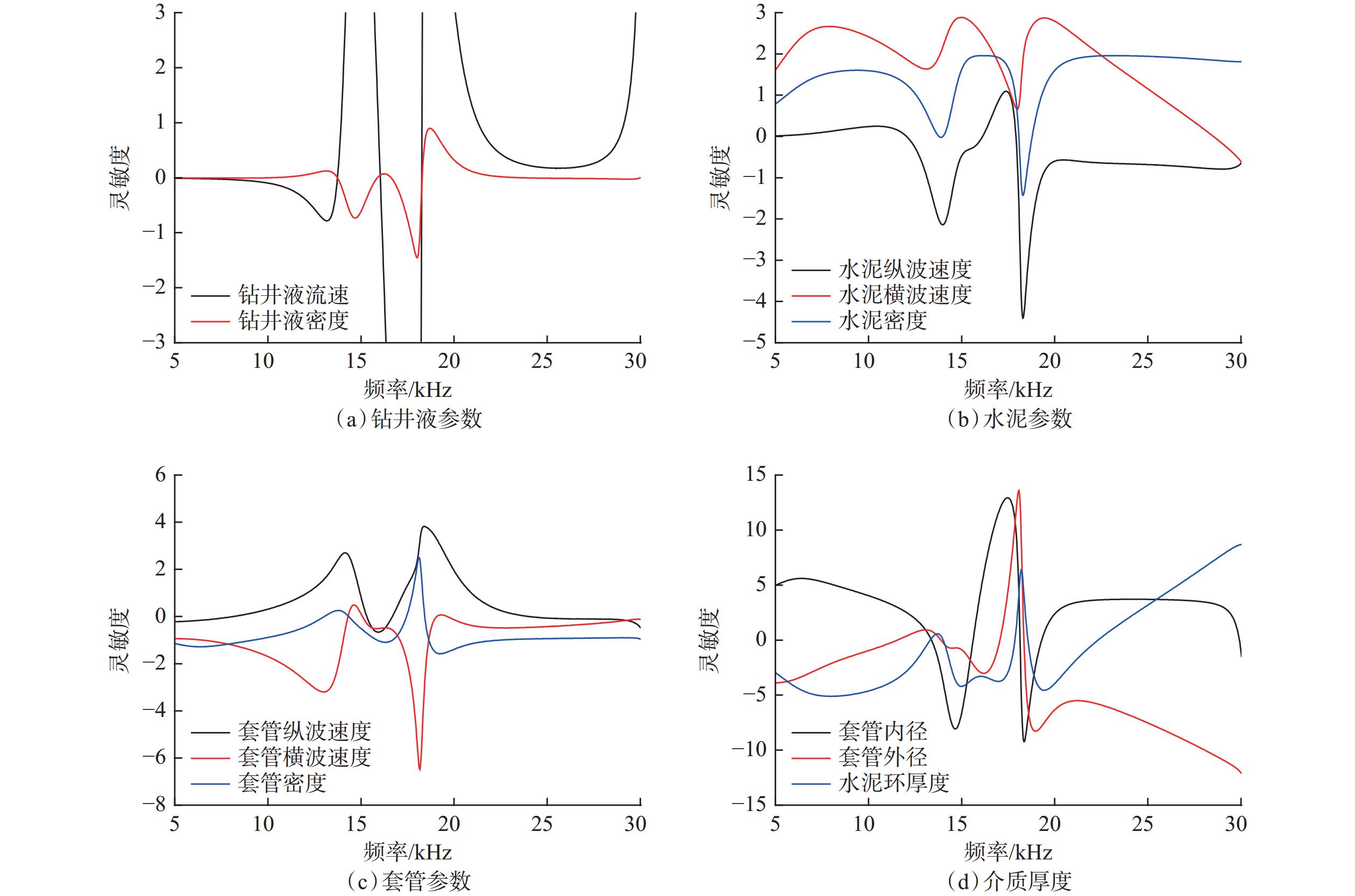
2.2 频散和衰减对套管和水泥各参数灵敏度的分析
套管波幅度或衰减作为评价固井质量的关键参数,除了受水泥胶结状况的影响外,对套管壁厚、水泥强度及水泥环厚度等均比较敏感,图6给出了套管波衰减对钻井液、套管及水泥等参数的灵敏度,水泥密度为1.30 kg/L。由图6(a)可知,井内钻井液声学参数对套管波衰减的影响较小。套管波的衰减更多依赖水泥环的横波速度,其次是水泥密度,见图6(b),可见在套管和水泥环界面能量的传递主要通过剪切耦合传递到水泥环和地层中,水泥的剪切模量越大,衰减越大。由图6(c)可知,套管材质的纵横波速度和密度对套管波衰减的影响也较大,但套管材质的纵横波速度和密度越大衰减越小。图6(d)所示套管和水泥环厚度发生变化时套管波衰减的灵敏度,在套管内径变大时,套管壁厚变小,灵敏度值是正值,说明套管管壁变薄套管波衰减增大;外径变大套管壁厚增厚,衰减减小,这与图3和图5的计算结果一致;特别是衰减对水泥环厚度的灵敏度也较高,随着水泥环厚度(水泥环外径)增大,衰减减小。
2.3 水泥环厚度对套管波的影响
从图6(d)可以看出,水泥环厚度对套管波的相速度和衰减影响也较大,这在几何声学上很难解释。G. H. Pardue等人[13]采用试验方法研究了水泥环厚度变化时套管模式波的衰减α,石油天然气行业标准《固井质量评价方法》(SY/T 6592—2016)[14]采用其试验结果,建立了套管波衰减的校正公式:
\alpha\mathrm{_c}=0.825\; 5d^{-0.538\; 5}\alpha (13) 式中:
\alpha\mathrm{_c} 为校正后的衰减值;\alpha 为校正前的衰减值,d为水泥环厚度。根据式(13)可知,水泥环厚度越小,校正后的衰减越大,即水泥环厚度变薄,会使测得套管波的幅度偏高[15],这与图6(d)的灵敏度计算结果不符。为了考察水泥环厚度对套管模式波幅度的影响,利用式(12)计算了水泥环第Ⅰ界面和第Ⅱ界面均胶结良好状态下套管波随着水泥环厚度变化的规律,结果如图7所示。从图7可以看出,套管外径139.7 mm保持不变,随着水泥环厚度从20.0 mm逐渐变为0,套管波幅度逐渐减小,即同样的胶结状况下,水泥环厚度较薄时测得套管波的幅度较低,因此当水泥环厚度校正为常规的19.0 mm时,套管波幅度会增大,这与图6(d)的计算结果一致。
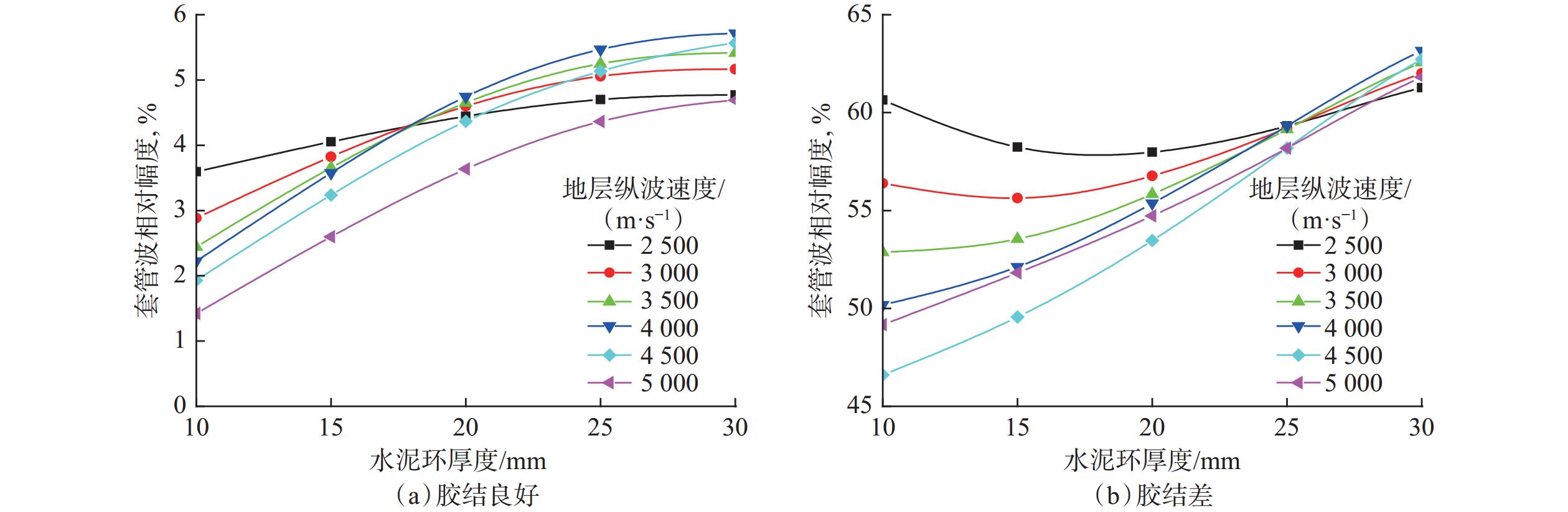
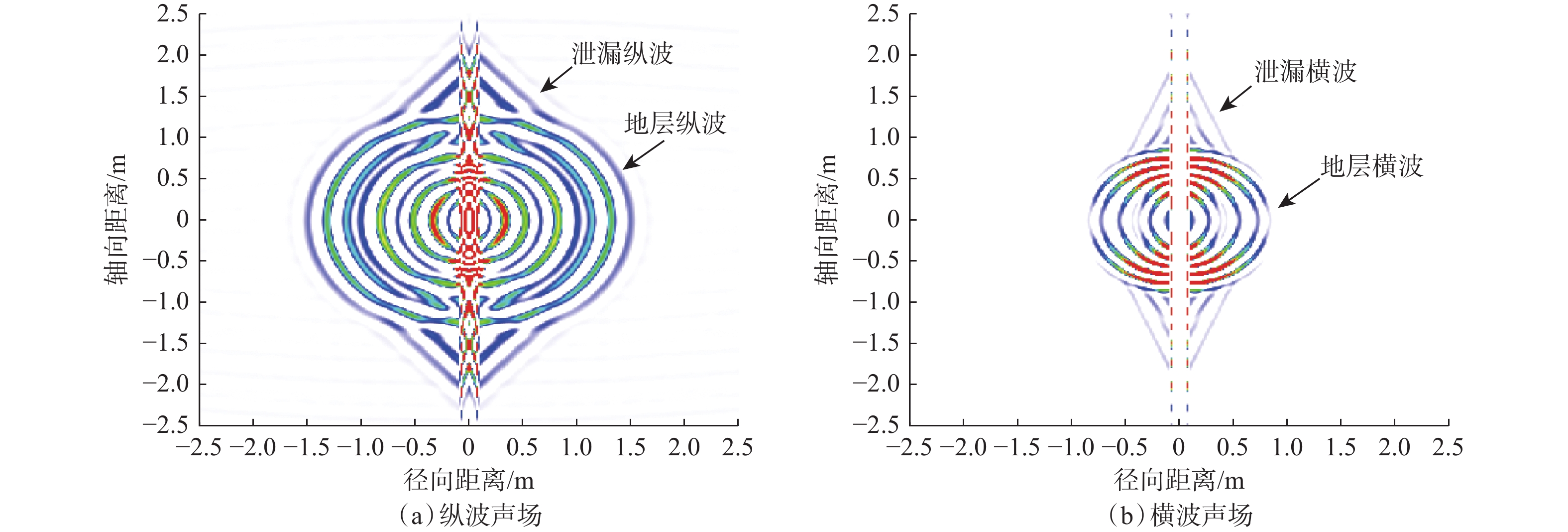
不同胶结状况下水泥环厚度变化时套管波相对幅度的变化趋势如图8所示。从图8可以看出,水泥胶结良好时,随着水泥环厚度变薄,套管波相对幅度逐渐降低;在速度较低的泥岩地层,若胶结较差(水泥环第Ⅰ界面有1.0 mm的流体环),随着水泥环厚度变薄,套管波相对幅度会出现先减小后增大的趋势;对于常规储层,地层速度越高,水泥环厚度变化引起套管波相对幅度的变化越大,基本随水泥环厚度变薄逐渐减小。图8展示的套管波幅度复杂的变化趋势,揭示了沿着套管传播的模式波(见图9),一边沿着套管传播,一边向其两侧介质泄漏声波能量[16–17],向水泥环和地层中泄漏的声波能量与界面的胶结情况、水泥环与地层声学性质及水泥环厚度等均有关系[18–19],在低速地层中,水泥环厚度变薄更有利于套管波泄漏能量,使衰减增大,幅度降低。
3. 结论与建议
1)影响套管波幅度的因素很多,但套管波幅度的高低主要取决于套后介质的横波速度,套管波衰减对水泥环横波速度的灵敏度明显高于纵波。随着横波速度增大,套管波衰减增大幅度减小;随着水泥环纵波速度增大,套管波幅度会稍有增大。
2)自由套管状态下,随着套管壁厚增大,套管波幅度基本不变,但套后胶结水泥时,随着套管壁厚增大,套管波能量会向套管内壁集中,使井内接收到套管波的幅度明显增大,且套后胶结水泥的阻抗越大,套管波幅度增大越明显。因此,现场应用时需要针对不同壁厚的套管,建立不同的固井质量解释图版。
3)套管波在沿着套管传播时还会向地层中泄漏声波能量,水泥环厚度及地层岩性变化均会导致能量的泄漏速度发生变化。因此,利用套管波幅度或衰减值校正水泥环厚度时,需要考虑岩性的影响。
-
表 1 套管井模型中各层介质的声学参数
Table 1 Acoustic parameters of each layer medium in cased well model
介质 纵波速度/(m·s−1) 横波速度/(m·s−1) 密度/(kg·m−3) 钻井液/水 1 500 1 000 套管 5 959 3 229 7 800 水泥Ⅰ 2 714 1 503 1 300 水泥Ⅱ 2 828 1 729 1 900 地层 3 500 2 000 2 500 -
[1] TUBMAN K M, CHENG C H, TOKSÖZ M N. Synthetic full waveform acoustic logs in cased boreholes[J]. Geophysics, 1984, 49(7): 1051–1059. doi: 10.1190/1.1441720
[2] 董庆德,王克协,许吉庆. 柱状多层准弹性介质中声压波形的数值计算与分析:声法测井理论研究(Ⅱ)[J]. 地球物理学报,1985,28(2):208–217. doi: 10.3321/j.issn:0001-5733.1985.02.010 DONG Qingde, WANG Kexie, XU Jiqing. Numerical evaluation and analysis of the acoustic pressure waveform in cylindrical multilayer quasi elastical media: a theoretical study of acoustic logging method (Ⅱ)[J]. Chinese Journal of Geophysics, 1985, 28(2): 208–217. doi: 10.3321/j.issn:0001-5733.1985.02.010
[3] 陈德华,王秀明,张海澜,等. 水泥密度和套管尺寸对油井套管波的影响[J]. 声学学报,2008,33(1):15–20. doi: 10.3321/j.issn:0371-0025.2008.01.003 CHEN Dehua, WANG Xiuming, ZHANG Hailan, et al. The effects of cement density and casing dimension on casing waves in oil wells[J]. Acta Acustica, 2008, 33(1): 15–20. doi: 10.3321/j.issn:0371-0025.2008.01.003
[4] ZHANG Xiumei, WANG Xiuming, ZHANG Hailan. Leaky modes and the first arrivals in cased boreholes with poorly bonded conditions[J]. Science China Physics, Mechanics & Astronomy, 2016, 59(2): 624301.
[5] WANG Hua, FEHLER M. The wavefield of acoustic logging in a cased-hole with a single casing: part I: a monopole tool[J]. Geophysical Journal International, 2018, 212(1): 612–626. doi: 10.1093/gji/ggx437
[6] LIU Yang, D’ANGELO R M, SINHA B K, et al. Acoustic guided waves in cylindrical solid-fluid structures: Modeling with a sweeping frequency finite element method and experimental validation[J]. AIP Conference Proceedings, 2017, 1806(1): 030004.
[7] 吴铭德,乔文孝,魏涛,等. 油气井封固性测井述评[J]. 测井技术,2016,40(1):1–11. WU Mingde, QIAO Wenxiao, WEI Tao, et al. Review on well integrity logging[J]. Well Logging Technology, 2016, 40(1): 1–11.
[8] 刘金璐,李军,柳贡慧,等. 深水固井循环阶段井筒温度场预测模型研究[J]. 石油钻探技术,2024,52(4):66–74. LIU Jinlu, LI Jun, LIU Gonghui, et al. Prediction model of wellbore temperature field during deepwater cementing circulation stage[J]. Petroleum Drilling Techniques, 2024, 52(4): 66–74.
[9] PONOMARENKO R, SABITOV D, CHARARA M. Spectral element simulation of elastic wave propagation through fractures using linear slip model: microfracture detection for CO2 storage[J]. Geophysical Journal International, 2020, 223(3): 1794–1804. doi: 10.1093/gji/ggaa399
[10] ZHANG Yanyan, FANG Xinding, GAN Yuandi. An efficient finite-difference approach for acoustic modeling in cased boreholes with micro-annuluses[R]. SEG 2019-3216563, 2019.
[11] 吴天乾,宋文宇,谭凌方,等. 超低密度水泥固井质量评价方法[J]. 石油钻探技术,2022,50(1):65–70. doi: 10.11911/syztjs.2021111 WU Tianqian, SONG Wenyu, TAN Lingfang, et al. Evaluation method for cementing quality of ultra-low-density cement[J]. Petroleum Drilling Techniques, 2022, 50(1): 65–70. doi: 10.11911/syztjs.2021111
[12] 谢关宝. 轻质水泥浆固井质量测井评价标准构建[J]. 石油钻探技术,2022,50(1):119–126. doi: 10.11911/syztjs.2022015 XIE Guanbao. Establishment of logging evaluation criteria for the cementing quality of low-density cement slurries[J]. Petroleum Drilling Techniques, 2022, 50(1): 119–126. doi: 10.11911/syztjs.2022015
[13] PARDUE G H, MORRIS R L. Cement bond log: a study of cement and casing variables[J]. Journal of Petroleum Technology, 1963, 15(5): 545–555. doi: 10.2118/453-PA
[14] SY/T 6592—2016 固井质量评价方法[S]. SY/T 6592—2016 Procedure for cement evaluation[S].
[15] 魏涛. 油气井固井质量测井评价[M]. 北京:石油工业出版社,2010:133-134. WEI Tao. Logging evaluation of cement bonding in cased boreholes[M]. Beijing: Petroleum Industry Press, 2010: 133-134.
[16] 潘金林,陈雪莲,唐晓明. 滑移界面表征的套管井声场的理论计算[J]. 地球物理学报,2023,66(1):442–453. doi: 10.6038/cjg2022P0832 PAN Jinlin, CHEN Xuelian, TANG Xiaoming. Theoretical calculation of casing well sound field represented by slip interface[J]. Chinese Journal of Geophysics, 2023, 66(1): 442–453. doi: 10.6038/cjg2022P0832
[17] 朱雷,陈雪莲,张鑫磊,等. 基于IBC和CBL/VDL测井的微间隙识别方法[J]. 石油钻探技术,2024,52(4):135–142. ZHU Lei, CHEN Xuelian, ZHANG Xinlei, et al. Identification method of microannulus based on IBC and CBL/VDL logging[J]. Petroleum Drilling Techniques, 2024, 52(4): 135–142.
[18] 李宁,刘鹏,范华军,等. 基于阵列声波测井的井下多尺度压裂效果评价方法[J]. 石油钻探技术,2024,52(1):1–7. LI Ning, LIU Peng, FAN Huajun, et al. Evaluation method of downhole multi-scale fracturing effect based on array acoustic logging[J]. Petroleum Drilling Techniques, 2024, 52(1): 1–7.
[19] 周仕明,陆沛青. 井筒密封完整性监测与智能感知技术进展与展望[J]. 石油钻探技术,2024,52(5):35–41. ZHOU Shiming, LU Peiqing. Advancements and prospects of monitoring and intelligent perception technology for wellbore sealing integrity[J]. Petroleum Drilling Techniques, 2024, 52(5): 35–41.



 下载:
下载: